Article for the “bio/mol/text” competition: Cellular processes that ensure the exchange of information between neurons require a lot of energy. High energy consumption has contributed to the selection of the most efficient mechanisms for encoding and transmitting information during evolution. In this article, you will learn about the theoretical approach to the study of brain energy, its role in the study of pathologies, which neurons are more advanced, why synapses sometimes benefit from not “firing,” and how they select only the information the neuron needs.
Origin of the approach
Since the mid-twentieth century, it has been known that the brain consumes a significant part of the energy resources of the whole organism: a quarter of all glucose and ⅕ of all oxygen in the case of the great ape [1–5]. This inspired William Levy and Robert Baxter from the Massachusetts Institute of Technology (USA) to conduct a theoretical analysis of the energy efficiency of information encoding in biological neural networks (Fig. 1) [6]. The study is based on the following hypothesis. Since the brain's energy consumption is high, it is beneficial for it to have neurons that work most efficiently - transmitting only useful information and expending a minimum of energy.
This assumption turned out to be true: using a simple neural network model, the authors reproduced the experimentally measured values of some parameters [6]. In particular, the optimal frequency of impulse generation they calculated varies from 6 to 43 impulses/s - almost the same as for neurons at the base of the hippocampus. They can be divided into two groups according to the pulse frequency: slow (~10 pulses/s) and fast (~40 pulses/s). Moreover, the first group significantly outnumbers the second [7]. A similar picture is observed in the cerebral cortex: there are several times more slow pyramidal neurons (~4-9 impulses/s) than fast inhibitory interneurons (>100 impulses/s) [8], [9]. Thus, apparently, the brain “prefers” to use fewer fast and energy-consuming neurons so that they do not use up all their resources [6], [9–11].
Figure 1. Two neurons are shown. In one of them, the presynaptic protein synaptophysin is colored purple. Another neuron is completely stained with green fluorescent protein. Small light specks are synaptic contacts between neurons [12]. In the inset, one “speck” is presented closer. Groups of neurons connected by synapses are called neural networks [13], [14]. For example, in the cerebral cortex, pyramidal neurons and interneurons form extensive networks. The coordinated “concert” work of these cells determines our higher cognitive and other abilities. Similar networks, only made up of different types of neurons, are distributed throughout the brain, connected in a certain way and organize the work of the entire organ.
website embryologie.uni-goettingen.de
What are interneurons?
Neurons of the central nervous system are divided into activating (forming activating synapses) and inhibitory (forming inhibitory synapses). The latter are largely represented by interneurons , or intermediate neurons. In the cerebral cortex and hippocampus, they are responsible for the formation of gamma rhythms in the brain [15], which ensure the coordinated, synchronous work of other neurons. This is extremely important for motor functions, perception of sensory information, and memory formation [9], [11].
Interneurons are distinguished by their ability to generate significantly higher frequency signals than other neurons. They also contain more mitochondria, the main organelles of energy metabolism and ATP production factories. The latter also contain a large amount of proteins cytochrome c oxidase and cytochrome c, which are key for metabolism. Thus, interneurons are extremely important and, at the same time, energy-consuming cells [8], [9], [11], [16].
The work of Levy and Baxter [6] develops the concept of “economy of impulses” by Horace Barlow from the University of California (USA), who, by the way, is a descendant of Charles Darwin [17]. According to it, during the development of the body, neurons tend to work only with the most useful information, filtering out “extra” impulses, unnecessary and redundant information. However, this concept does not provide satisfactory results, since it does not take into account the metabolic costs associated with neuronal activity [6]. Levy and Baxter's extended approach, which focuses on both factors, has been more fruitful [6], [18–20]. Both the energy expenditure of neurons and the need to encode only useful information are important factors guiding brain evolution [6], [21–24]. Therefore, in order to better understand how the brain works, it is worth considering both of these characteristics: how much useful information a neuron transmits and how much energy it spends.
Recently, this approach has found many confirmations [10], [22], [24–26]. It allowed us to take a new look at the structure of the brain at various levels of organization - from molecular biophysical [20], [26] to organ [23]. It helps to understand what the trade-offs are between the function of a neuron and its energy cost and to what extent they are expressed.
How does this approach work?
Suppose we have a model of a neuron that describes its electrophysiological properties: action potential ( AP ) and postsynaptic potentials ( PSP ) (more on these terms below). We want to understand whether it works efficiently and whether it wastes an unreasonable amount of energy. To do this, it is necessary to calculate the values of the model parameters (for example, the density of channels in the membrane, the speed of their opening and closing), at which: (a) the maximum ratio of useful information to energy consumption is achieved and at the same time (b) realistic characteristics of the transmitted signals are preserved [6 ], [19].
Search for the optimum
In fact, we are talking about an optimization problem: finding the maximum of a function and determining the parameters under which it is achieved. In our case, the function is the ratio of the amount of useful information to energy costs. The amount of useful information can be approximately calculated using Shannon's formula, widely used in information theory [6], [18], [19]. There are two methods for calculating energy costs, and both give plausible results [10], [27]. One of them - the “ion counting method” - is based on counting the number of Na+ ions that entered the neuron during a particular signaling event (AP or PSP, see sidebar “What is an action potential”) with subsequent conversion to the number of adenosine triphosphate (ATP) molecules ), the main energy “currency” of cells [10]. The second is based on the description of ionic currents through the membrane according to the laws of electronics and allows one to calculate the power of the equivalent electrical circuit of a neuron, which is then converted into ATP costs [17].
These “optimal” parameter values must then be compared with those measured experimentally to determine how different they are. The overall picture of the differences will indicate the degree of optimization of a given neuron as a whole: how real, experimentally measured, parameter values coincide with the calculated ones. The less pronounced the differences, the closer the neuron is to the optimum and the more energetically it works optimally. On the other hand, a comparison of specific parameters will show in what specific quality this neuron is close to the “ideal”.
Next, in the context of the energetic efficiency of neurons, two processes on which the encoding and transmission of information in the brain are based are considered. This is a nerve impulse, or action potential, thanks to which information can be sent to the “addressee” over a certain distance (from micrometers to one and a half meters) and synaptic transmission, which underlies the actual transmission of a signal from one neuron to another.
Speed of propagation of nerve impulses
In 1830, one of the greatest physiologists of the 19th century, Johann Muller, stated that the speed of propagation of PD was impossible to measure. In his opinion, since PD is an electrical impulse, it should be carried out at a speed approximately equal to the speed of light (3–1010 cm/s); Given the small size of biological objects, even with the best instruments of the time it was impossible to measure such a speed.
15 years later, one of Müller’s students, Hermann von Helmholtz, using a simple and elegant experiment that can be easily reproduced in a student laboratory workshop (Fig. 6–8), measured the speed of propagation of impulses in the nerve of a frog. In his experiments, Helmholtz irritated the nerve in two areas spaced 3 cm apart and measured the time from the moment the stimulus was given to the maximum muscle contraction. Let's assume that when the distal (located closer to the muscles) area is irritated, this time decreases by 1 ms. Then the pulse propagation speed V
equal to
| V | = | d | / | t | = | 3 cm | / | 1 ms | = | · | 103 | cm/s |
| Rice. 6.8. An experimental setup similar to the one with which Helmholtz measured the speed of propagation of impulses in the frog nerve. The stimulating electrodes were first applied to point St1 and then to point St2. A lever was connected to the muscle, the pointed end of which drew a curve on a sooty sheet of paper, which was quickly moved in the longitudinal direction. |
This value turned out to be seven orders of magnitude less than the speed of propagation of electric current in a copper conductor or in an electrolyte solution. From this, Helmholtz made the absolutely correct conclusion that the conduction of a nerve impulse is a more complex process than the simple longitudinal propagation of current in a nerve fiber.
The speed of impulse propagation in different axons varies from 120 m/s (in some large fibers) to several centimeters per second (in very thin axons). These differences between the conduction speed in different fibers are illustrated in Table. 6–1 and fig. 6–9.
The speed of impulse propagation largely depends on how quickly a section of the membrane located at a certain distance from the site of stimulus delivery is depolarized by local currents to a threshold level. The higher the fiber length constant, the further these currents can propagate, the faster the depolarization of the membrane in front of the excitation site occurs and, therefore, the higher the speed of impulse propagation. The effect of constant length on this speed can be demonstrated by placing the axon in oil or air. In this case, only a thin film of saline solution remains on the surface of the axon, and the length constant decreases due to an increase in the external longitudinal resistance [in equation (6–2) – r
0]. Under these conditions, the speed of excitation will be lower than when the axon is immersed in a saline solution.
Table 6-1. Classification of frog nerve fibers according to their diameter and speed of excitation (Erlanger, Gasser, 1937)
| Fiber group | Diameter, microns | Speed, m/s |
| A α | 18,5 | |
| β | 14,0 | |
| γ | 11,0 | |
| B | — | 4,2 |
| C | 2,5 | 0,4 –0,5 |
| Rice. 6.9. The speed of propagation of excitation in various groups of frog nerve fibers. A. Experimental setup for stimulating a bundle of nerve fibers and recording the resulting potentials. B. Composite action potential recorded using extracellular electrodes and representing the sum of potentials in all excited fibers of the bundle. Group α fibers have the largest diameter and are characterized by the highest conduction speed. In contrast, group γ fibers have both the diameter and conduction velocity the lowest (see Table 6-1). Stimulation was carried out until the start of recording. |
In the process of evolution, living organisms have developed two ways to increase the constant length of the axon and thereby the speed of impulse propagation. One of them (a typical example would be giant axons of squids, arthropods, annelids, and teleost fishes) is an increase in the diameter of the axon, i.e., a decrease in the internal longitudinal resistance [in equation (6–2) – r
i] This issue is discussed in more detail in Appendix 6-2. Giant axons have evolved in some animal species to provide rapid synchronous activation of motor reflexes, such as mantle movements in squid and the withdrawal or avoidance reflex in some arthropods (crayfish, cockroaches) and annelids (e.g., earthworms).
Action potential
Action potential ( AP ) is a signal that neurons send to each other. APs can be different: fast and slow, small and large [28]. They are often organized in long sequences (like letters in words) or in short, high-frequency “packs” (Fig. 2).
Figure 2. Different types of neurons generate different signals. In the center is a longitudinal section of the mammal's brain. The boxes show different types of signals recorded by electrophysiological methods [15], [38]. a — Cortical (Cerebral cortex) pyramidal neurons can transmit both low-frequency signals (Regular firing) and short explosive, or burst, signals (Burst firing). b - Purkinje cells of the cerebellum (Cerebellum) are characterized only by burst activity at a very high frequency. c — Relay neurons of the thalamus (Thalamus) have two modes of activity: burst and tonic (Tonic firing). d — Neurons in the middle part of the leash (MHb, Medial habenula) of the epithalamus generate low-frequency tonic signals.
[14], figure adapted
The wide variety of signals is due to the huge number of combinations of different types of ion channels, synaptic contacts, as well as the morphology of neurons [28], [29]. Since neuronal signaling processes are based on ionic currents, it is expected that different APs require different energy inputs [20], [27], [30].
What is an action potential?
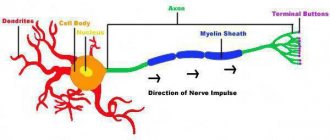
- Membrane and ions. The plasma membrane of a neuron maintains an uneven distribution of substances between the cell and the extracellular environment (Fig. 3b) [31–33]. Among these substances there are also small ions, of which K+ and Na+ are important for describing PD. There are few Na+ ions inside the cell, but many outside. Because of this, they constantly strive to get into the cage. On the contrary, there are a lot of K+ ions inside the cell, and they strive to leave it. The ions cannot do this on their own, because the membrane is impermeable to them. For ions to pass through the membrane, it is necessary to open special proteins—membrane ion channels.
- Ion channels. The variety of channels is enormous [14], [36], [38], [39]. Some open in response to a change in membrane potential, others - upon binding of a ligand (a neurotransmitter in a synapse, for example), others - as a result of mechanical changes in the membrane, etc. Opening a channel involves changing its structure, as a result of which ions can pass through it. Some channels allow only a certain type of ion to pass through, while others are characterized by mixed conductivity. In the generation of AP, a key role is played by channels that “sense” the membrane potential—voltage-dependent ion channels. They open in response to changes in membrane potential. Among them, we are interested in voltage-gated sodium channels (Na channels), which allow only Na+ ions to pass through, and voltage-gated potassium channels (K-channels), which allow only K+ ions to pass through.
- Ion current and PD. The basis of AP is the ion current—the movement of ions through the ion channels of the membrane [38]. Since the ions are charged, their current leads to a change in the net charge inside and outside the neuron, which immediately entails a change in the membrane potential. Generation of APs, as a rule, occurs in the initial segment of the axon—in that part that is adjacent to the neuron body [40], [14]. Many Na channels are concentrated here. If they open, a powerful current of Na+ ions will flow into the axon, and membrane depolarization will occur—a decrease in membrane potential in absolute value (Fig. 3c). Next, it is necessary to return to its original meaning - repolarization. K+ ions are responsible for this. When the K channels open (shortly before the AP maximum), K+ ions will begin to leave the cell and repolarize the membrane. Depolarization and repolarization are the two main phases of AP. In addition to them, there are several more, which, due to lack of necessity, are not considered here. A detailed description of PD generation can be found in [14], [29], [38], [41]. A brief description of PD is also available in articles on Biomolecule [15], [42].
- Initial axon segment and AP initiation. What causes Na channels to open at the axon initial segment? Again, a change in membrane potential “coming” along the dendrites of the neuron (Fig. 3a). These are postsynaptic potentials (PSPs) that arise as a result of synaptic transmission. This process is explained in more detail in the main text.
- Conducting PD. Na-channels located nearby will be indifferent to the AP in the initial segment of the axon. They too will open in response to this change in membrane potential, which will also cause AP. The latter, in turn, will cause a similar “reaction” on the next section of the axon, further and further from the neuron body, and so on. In this way, AP conduction occurs along the axon [14], [15], [38]. Eventually it will reach its presynaptic terminals (magenta arrows in Fig. 3a), where it can cause synaptic transmission.
- Energy consumption for the generation of APs is less than for the operation of synapses. How many molecules of adenosine triphosphate (ATP), the main energy “currency”, does PD cost? According to one estimate, for pyramidal neurons of the rat cerebral cortex, the energy consumption for generating 4 APs per second is about ⅕ of the total energy consumption of the neuron. If we take into account other signaling processes, in particular synaptic transmission, the share will be ⅘. For the cerebellar cortex, which is responsible for motor functions, the situation is similar: the energy consumption for generating the output signal is 15% of the total, and about half is for processing input information [25]. Thus, PD is far from the most energy-intensive process. The operation of a synapse requires many times more energy [5], [19], [25]. However, this does not mean that the PD generation process does not exhibit energy efficiency features.
Figure 3. Neuron, ion channels and action potential. a — Reconstruction of a candelabra cell in the rat cerebral cortex. The dendrites and body of the neuron are colored blue (blue spot in the center), the axon is colored red (in many types of neurons the axon is branched much more than the dendrites [8], [11], [35]). Green and crimson arrows indicate the direction of information flow: the dendrites and body of the neuron receive it, the axon sends it to other neurons. b - The membrane of a neuron, like any other cell, contains ion channels. Green circles are Na+ ions, blue circles are K+ ions. c — Change in membrane potential during the generation of an action potential (AP) by a Purkinje neuron. Green area: Na channels are open, Na+ ions enter the neuron, depolarization occurs. Blue area: K channels are open, K+ comes out, repolarization occurs. The overlap of the green and blue regions corresponds to the period when simultaneous entry of Na+ and exit of K+ occurs.
[34], [36], [37], figures adapted
AP is a relatively strong in amplitude stepwise change in membrane potential.
Analysis of different types of neurons (Fig. 4) showed that invertebrate neurons are not very energy efficient, while some vertebrate neurons are almost perfect [20]. According to the results of this study, the most energy efficient were the interneurons of the hippocampus, which is involved in the formation of memory and emotions, as well as thalamocortical relay neurons, which carry the main flow of sensory information from the thalamus to the cerebral cortex.
Figure 4. Different neurons are efficient in different ways. The figure shows a comparison of the energy consumption of different types of neurons. Energy consumption is calculated in models with both initial (real) parameter values (black columns) and optimal ones, in which, on the one hand, the neuron performs its assigned function, and on the other, it spends a minimum of energy (gray columns). The most effective of the presented ones turned out to be two types of vertebrate neurons: hippocampal interneurons (rat hippocampal interneuron, RHI) and thalamocortical neurons (mouse thalamocortical relay cell, MTCR), since for them the energy consumption in the original model is closest to the energy consumption of the optimized one. In contrast, invertebrate neurons are less efficient. Legend: SA (squid axon) - squid giant axon; CA (crab axon) - crab axon; MFS (mouse fast spiking cortical interneuron) - fast cortical interneuron of the mouse; BK (honeybee mushroom body Kenyon cell) - mushroom-shaped Kenyon cell of a bee.
[20], figure adapted
Why are they more effective? Because they have little overlap of Na- and K-currents. During the generation of APs, there is always a period of time when these currents are present simultaneously (Fig. 3c). In this case, practically no charge transfer occurs, and the change in membrane potential is minimal. But in any case, you have to “pay” for these currents, despite their “uselessness” during this period. Therefore, its duration determines how much energy resources are wasted. The shorter it is, the more efficient the energy use [20], [26], [30], [43]. The longer, the less effective. In just the two above-mentioned types of neurons, thanks to fast ion channels, this period is very short, and APs are the most effective [20].
By the way, interneurons are much more active than most other neurons in the brain. At the same time, they are extremely important for the coordinated, synchronous operation of neurons, with which they form small local networks [9], [16]. Probably, the high energy efficiency of AP interneurons is some kind of adaptation to their high activity and role in coordinating the work of other neurons [20].
Saltatory conduction
The second way to increase the speed of nerve impulses, realized only in vertebrates, is to isolate sections of the axon using the myelin sheath.
In this case, the length constant of the corresponding sections increases significantly, and thereby the conduction of current in the longitudinal direction is significantly facilitated. As the animal develops, myelin is deposited around peripheral and central axons by glial cells located near these axons. As a result, a dense multilayer shell of cell membranes is formed around the fibers.
Cells that synthesize myelin include Schwann cells
(in the area of peripheral nerves) and
oligodendrocytes
(Fig. 6–10) (in the central nervous system).
Transverse sections of the myelin sheath show periodically repeating gaps of 12 nm, resulting from the layering of glial cell membranes. With the formation of each new layer, the lateral resistance of the shell increases. Since there are many layers in this shell, its capacity is much lower than that of a single membrane. The multilayer myelin sheath is periodically interrupted (the so-called nodes of Ranvier},
and in these small areas the excitable axon membrane is in contact with the extracellular environment. Between the nodes of Ranvier, the myelin sheath is closely adjacent to the axon membrane, practically displacing the extracellular environment. In addition, sections of the axon membrane between the nodes of Ranvier , apparently, do not contain sodium channels.
| Rice. 6.10. Interception of Ranvier. Shown is a short "bare" section of an axon located between two myelinated regions. It is this area that is excited during saltatory conduction. In Fig. 4–12 shows an electron micrograph showing a multilayer myelin sheath formed by the membranes of glial cells. (Bunge et al., 1961.) |
Due to the insulating properties of the myelin sheath, the axon length constant increases sharply: the presence of this sheath has the same effect as an increase in r
m [Equation (6–2)]. Due to the high resistance of the myelin sheath, local currents flowing ahead of the excitation wave exit the axon almost exclusively in the region of the nodes of Ranvier. In addition, since the capacitance of the thick myelin sheath is small, only a very small current is consumed to recharge this capacitance in the areas between nodes.
Due to these features, the AP arising in any interception electrotonically depolarizes only the membrane located in the area of the next interception, and therefore impulses in such axons do not propagate along their entire length, as in unmyelinated nerve fibers (for example, in the squid axon). They arise only in small areas of the membrane—the nodes of Ranvier. All this causes saltatory
(spasmodic)
conduction,
in which impulses propagate intermittently from interception to interception (Fig. 6–11). Speed of spread. In this case, the PD increases sharply, since the electrotonic conduction of local currents between interceptions occurs very quickly. Thus, in vertebrates, Nature solved the problem of the rapid propagation of nerve impulses without resorting to the creation of such bulky structures as giant axons.
Synapse
The transmission of a signal from one neuron to another occurs in a special contact between neurons, in the synapse [12]. We will consider only chemical synapses (there are also electrical ones), since they are very common in the nervous system and are important for the regulation of cellular metabolism and nutrient delivery [5].
Most often, a chemical synapse is formed between the axon terminal of one neuron and the dendrite of another. His work resembles... “relaying” a relay baton, the role of which is played by a neurotransmitter - a chemical mediator of signal transmission [12], [42], [44–48].
At the presynaptic end of the axon, the AP causes the release of a neurotransmitter into the extracellular environment - to the receiving neuron. The latter is looking forward to just this: in the membrane of the dendrites, receptors - ion channels of a certain type - bind the neurotransmitter, open and allow different ions to pass through them. This leads to the generation of a small postsynaptic potential (PSP) on the dendrite membrane. It resembles AP, but is much smaller in amplitude and occurs due to the opening of other channels. Many of these small PSPs, each from its own synapse, “run” along the dendrite membrane to the neuron body (green arrows in Fig. 3a) and reach the initial segment of the axon, where they cause the opening of Na channels and “provoke” it to generate APs.
Such synapses are called excitatory : they contribute to the activation of the neuron and the generation of AP. There are also inhibitory synapses. They, on the contrary, promote inhibition and prevent the generation of AP. Often one neuron has both synapses. A certain ratio between inhibition and excitation is important for normal brain function and the formation of brain rhythms that accompany higher cognitive functions [49].
Oddly enough, the release of a neurotransmitter at the synapse may not occur at all - this is a probabilistic process [18], [19]. Neurons save energy in this way: synaptic transmission already accounts for about half of all energy expenditure of neurons [25]. If synapses always fired, all the energy would go into keeping them functioning, and there would be no resources left for other processes. Moreover, it is the low probability (20–40%) of neurotransmitter release that corresponds to the highest energetic efficiency of synapses. The ratio of the amount of useful information to the energy expended in this case is maximum [18], [19]. So, it turns out that “failures” play an important role in the functioning of synapses and, accordingly, the entire brain. And you don’t have to worry about signal transmission when synapses sometimes don’t work, since there are usually many synapses between neurons, and at least one of them will work.
Another feature of synaptic transmission is the division of the general flow of information into individual components according to the modulation frequency of the incoming signal (roughly speaking, the frequency of incoming APs) [50]. This occurs due to the combination of different receptors on the postsynaptic membrane [38], [50]. Some receptors are activated very quickly: for example, AMPA receptors (AMPA comes from α- a mino-3-hydroxy-5- m ethyl-4-isoxazole p ropionic acid ). If only such receptors are present on the postsynaptic neuron, it can clearly perceive a high-frequency signal (such as, for example, in Fig. 2c). The most striking example is the neurons of the auditory system, which are involved in determining the location of a sound source and accurately recognizing short sounds such as clicks, which are widely represented in speech [12], [38], [51]. NMDA receptors (NMDA - from N - m ethyl- D - a spartate) are slower. They allow neurons to select signals of lower frequency (Fig. 2d), as well as to perceive a high-frequency series of APs as something unified—the so-called integration of synaptic signals [14]. There are even slower metabotropic receptors, which, when binding a neurotransmitter, transmit a signal to a chain of intracellular “second messengers” to adjust a wide variety of cellular processes. For example, G protein-associated receptors are widespread. Depending on the type, they, for example, regulate the number of channels in the membrane or directly modulate their operation [14].
Various combinations of fast AMPA, slower NMDA, and metabotropic receptors allow neurons to select and use the most useful information that is important for their functioning [50]. And “useless” information is eliminated; it is not “perceived” by the neuron. In this case, you don’t have to waste energy processing unnecessary information. This is another aspect of optimizing synaptic transmission between neurons.
Nerve impulse transmission speed
Nerve impulse, propagation of excitation (bioelectric impulse) along nerve fibers in response to irritation of neurons.
In the second half of the 19th century, in the work of G. Helmholtz and E. Hering on the frog nerve, it was shown that the bioelectric signal (current, or action potential), unlike the electric current in a conventional conductor, propagates along the nerve fiber with a finite speed (3- 120 m/sec).
The possibility of propagation of nerve impulses along nerve fibers is determined by their structure, which is reminiscent of the structure of an electric cable, where the role of a conductor is played by axons, and the role of an insulator is the axon’s myelin sheath, which is a Schwann cell membrane wound around the axon in several layers.
The main component of the myelin sheath is the lipoprotein myelin, which has dielectric properties. The speed of propagation of nerve impulses depends both on the diameter of the nerve fibers (the thicker the fiber, the higher the speed), and on the degree of their electrical insulation, since myelin-coated fibers, other things being equal, conduct nerve impulses faster. The myelin sheath does not cover the fiber continuously along its entire length, but forms a kind of insulating ceramic “couplings” tightly strung on the axon, like on the rod of an electrical cable.
Between adjacent “couplings” of myelin, only small electrically non-insulated areas remain, through which ionic current can easily flow from the axon into the external environment and back, irritating the membrane and causing the generation of an action potential exclusively in non-insulated areas of the axon, called nodes of Ranvier. The nerve impulse spreads along the myelinated nerve fiber in jumps - from one node of Ranvier to the next, which significantly increases the speed of propagation of excitation from cell to cell.
The speed of propagation of a nerve impulse along thick myelinated fibers (10-20 microns in diameter) in humans reaches 70-120 m/sec, and along the thinnest unmyelinated nerve fibers it is two orders of magnitude lower (less than 2 m/sec).
The ability to produce nerve impulses is one of the fundamental properties of neurons.
Nerve impulses provide rapid transmission of similar signals (action potentials) along axons over long distances and therefore are the most important means of exchanging information both between nerve cells and between nerve and other types of cells. Information about the strength of stimulation of a nerve cell is encoded and transmitted to other cells by changing the frequency of nerve impulses.
The repetition rate can vary from a few to hundreds of nerve impulses per second. The frequency code assumes a complex periodicity of nerve impulses, including their grouping into “packs” with different numbers and patterns of signals. The complex spatial and temporal summation of nerve impulses forms the basis of the rhythmic electrical activity of the brain, recorded using an electroencephalogram.
The speed of propagation of nerve impulses can vary: less than 1 meter per second in very thin axons and about 100 meters per second in thick axons (for example, in axons innervating muscles).
An electrical impulse propagating along the axon, reaching the axon endings on another nerve cell, suddenly disappears. Charles Sherrington, who laid the foundations of the so-called synaptology, called the points of contact between the endings of an axon and another nerve cell “synapses.”
In order to “cross” a synapse, the nerve impulse must be regenerated on the other side of the synapse. Even 15 years ago, some physiologists believed that the transmission of impulses through a synapse is a phenomenon mainly (if not entirely) of an electrical order. Now, however, there is ample evidence that during such transmission there is a release of special substances that cause impulse regeneration. The first convincing evidence that a transmitter substance operates in a synapse was obtained more than 40 years ago by G. Dale and O. Löwy.
As is known, the human central nervous system (including, of course, not only the brain, but also the spinal cord) consists of approximately 10 billion (1010) nerve cells. Almost all nerve cells, with rare exceptions, receive information directly in the form of impulses (see figure below) from several nerve cells at once (often hundreds of them) and transmit it to an equally large number of cells.
What else?
The energy efficiency of brain cells has also been studied in relation to their morphology [35], [52–54]. Studies show that the branching of dendrites and axons is not chaotic and also saves energy [52], [54]. For example, an axon branches so that the total length of the path that passes through the AP is minimal. In this case, the energy consumption for conducting AP along the axon is minimal.
A reduction in neuron energy consumption is also achieved at a certain ratio of inhibitory and excitatory synapses [55]. This is directly related, for example, to ischemia (a pathological condition caused by impaired blood flow in the vessels) of the brain. In this pathology, the most metabolically active neurons are most likely to fail first [9], [16]. In the cortex, they are represented by inhibitory interneurons that form inhibitory synapses on many other pyramidal neurons [9], [16], [49]. As a result of the death of interneurons, the inhibition of pyramidal neurons decreases. As a consequence, the overall level of activity of the latter increases (activating synapses fire more often, APs are generated more often). This is immediately followed by an increase in their energy consumption, which under ischemic conditions can lead to the death of neurons.
When studying pathologies, attention is paid to synaptic transmission as the most energy-consuming process [19]. For example, in Parkinson's [56], Huntington's [57], and Alzheimer's diseases [58–61], there is a disruption in the functioning or transport to the synapses of mitochondria, which play a major role in the synthesis of ATP [62], [63]. In the case of Parkinson's disease, this may be due to disruption and death of highly energy-consuming neurons of the substantia nigra, which is important for the regulation of motor functions and muscle tone. In Huntington's disease, the mutant protein huntingtin disrupts the delivery mechanisms of new mitochondria to synapses, which leads to “energy starvation” of the latter, increased vulnerability of neurons and excessive activation. All this can cause further disruption of neuronal function with subsequent atrophy of the striatum and cerebral cortex. In Alzheimer's disease, mitochondrial dysfunction (in parallel with a decrease in the number of synapses) occurs due to the deposition of amyloid plaques. The effect of the latter on mitochondria leads to oxidative stress, as well as apoptosis - cell death of neurons.
Excitation and inhibition of a nerve cell
Excitation and inhibition of a nerve cell are carried out by nerve fibers that form synapses on its surface.
Top (1) motor neuron at rest. Impulses arriving along one excitatory fiber (2) are not yet able to cause a discharge of the motor neuron. A discharge occurs only when impulses also arrive along the second excitatory fiber (3) (threshold state of the neuron). If the neuron also receives impulses along the inhibitory fiber, then it returns to the subthreshold state (4).
Bottom (b) - impulses arrive only along the inhibitory fiber. Electrical impulses propagating along excitatory and inhibitory nerve fibers do not differ from each other. Their opposite action is explained by the release of different chemical transmitters in the synaptic endings.
In a given nerve cell, depending on its excitation threshold, a discharge of impulses may occur when only a few fibers coming to it are irritated; in other cases, the discharge of impulses does not occur even when many such fibers are irritated.
It has long been known that various factors can increase or decrease the threshold of excitation of a nerve cell.
Moreover, about 60 years ago it was suggested that some fibers should inhibit the discharge of impulses in the cell to which they approach, rather than excite it. This assumption was subsequently confirmed, and at present the mechanism of inhibition is clarified. This article is devoted to two equivalent processes—inhibition and its antipode—excitation of a nerve cell.
Once again about everything
At the end of the twentieth century, an approach to studying the brain emerged that simultaneously considers two important characteristics: how much a neuron (or neural network, or synapse) encodes and transmits useful information and how much energy it spends [6], [18], [19] . Their ratio is a kind of criterion for the energy efficiency of neurons, neural networks and synapses.
The use of this criterion in computational neuroscience has provided a significant increase in knowledge regarding the role of certain phenomena and processes [6], [18–20], [26], [30], [43], [55]. In particular, the low probability of neurotransmitter release at the synapse [18], [19], a certain balance between inhibition and excitation of a neuron [55], and the selection of only a certain type of incoming information due to a certain combination of receptors [50] - all this helps to save valuable energy resources.
Moreover, the very determination of the energy consumption of signaling processes (for example, generation, conduction of action potentials, synaptic transmission) makes it possible to find out which of them will suffer first in case of pathological disruption of nutrient delivery [10], [25], [56]. Since synapses require the most energy to operate, they are the first to fail in pathologies such as ischemia, Alzheimer’s and Huntington’s diseases [19], [25]. In a similar way, determining the energy consumption of different types of neurons helps to determine which of them will die before others in the event of pathology. For example, with the same ischemia, the interneurons of the cortex will fail first [9], [16]. Due to their intense metabolism, these same neurons are the most vulnerable cells during aging, Alzheimer’s disease, and schizophrenia [16].
In general, the approach to determining energetically efficient mechanisms of brain function is a powerful direction for the development of both fundamental neuroscience and its medical aspects [5], [14], [16], [20], [26], [55], [64]. ].
Content:
- 1 Membrane potential
- 2 Impulse
- 3 Synaptic transmission
- 4 Typical neural pathway
Description
Much of neuroscience
comprise sections on how individual neurons work and how information is passed from cell to cell through synapses. It should be obvious that without this information we would be in the position of someone who wants to understand the operation of a radio or television, but knows nothing about resistors, capacitors and transistors. Over the past decades, thanks to the ingenuity of a number of neurophysiologists, the most famous of whom are Andrew Huxley, Alan Hodgkin, Bernard Katz, John Eccles and Stephen Kuffler, the physicochemical mechanisms of nerve impulses and synaptic transmission have been well studied. However, it is equally obvious that information of this kind in itself cannot yet lead to an understanding of the functioning of the brain, just as knowledge about resistors, capacitors and transistors alone will not allow one to understand the operation of a radio or television, and knowledge of the chemistry of ink will not allow one to read a Shakespeare play.
I begin this article by summarizing some of what we know about nerve conduction and synaptic transmission.
Knowledge of the fundamentals of physical chemistry and electricity will be a great help in a correct understanding of the essence of the matter, but I think that even without this the reader will be able to get a sufficient understanding of the subject. In any case, you will need only a rudimentary understanding of these issues to follow the discussion in subsequent chapters.
The task of the nerve cell
is to receive information from the cells that transmit it, summarize, or integrate, this information and deliver the integrated information to other cells. Information is usually transmitted in the form of short-term processes called nerve impulses. In any cell, each impulse is exactly the same as any other, that is, an impulse is a stereotypical process. At any moment, the frequency of impulses sent by a neuron is determined by the signals it has just received from transmitting cells and transmits information to the cells for which this neuron is transmitting. The frequency of the pulses varies from one every few seconds or even lower to a maximum of about a thousand per second.
↑ Membrane potential
What happens when information is transferred from one cell to another through a synapse?
In the first - presynaptic - cell near the base of the axon, an electrical signal, or impulse, appears. The impulse moves along the axon to its endings. From each ending, as a result of this impulse, a chemical substance is released into the narrow (0.02 μm) fluid-filled gap separating one cell from another - the synaptic cleft - which diffuses to the second - postsynaptic - cell. It affects the membrane of this second cell in such a way that the probability of impulses occurring in it either decreases or increases. After this brief description, let's go back and look at the whole process in detail.
Rice.
8. Diagram of the arrangement of nerve cells on a transverse section of the retina, drawn by Santiago Ramon y Cajal, the greatest neuroanatomist of all time. From the top layer, where the thinner rods and thicker cones are shown, to the bottom layer, where the optic nerve fibers exit to the right, the retina is a quarter of a millimeter thick.
The nerve cell is washed with a saline solution and contains it inside. The salts include not only sodium chloride, but also potassium chloride, calcium chloride and a number of other, less common salts. Since most salt molecules are dissociated, fluids both inside and outside the cell contain chlorine, potassium, sodium, and calcium ions (Cl-, K+, Na+, and Ca2+).
At rest, the electrical potentials inside and outside the cell differ by about one tenth of a volt, with the plus being on the outside. The exact value is closer to 0.07 volts, or 70 millivolts. The signals transmitted by nerves are rapid changes in potential that travel along the fiber from the cell body to the axon terminals. I'll start by describing how a potential difference occurs across the cell membrane.
Rice.
9. In this electron micrograph (a section of the rat cerebellar cortex), the synapse appears as a narrow dark stripe at the bottom of the picture in the middle. To the left of the synapse, you can see a cross-section of an axon filled with tiny round synaptic vesicles that store the neurotransmitter. To the right of the synapse, a dendrite projection (called a spine) is visible; it extends from a large dendritic branch located horizontally at the top of the picture (the two dark sausage-shaped structures in this dendrite are mitochondria). At the synapse, two membranes are brought together - the membranes of the axon and the dendrite; here they are thickened and look more dense. They are separated by a gap 20 nanometers wide.
Nerve cell membrane covering the entire neuron
, - the structure is extremely complex. It is not solid, like a balloon or a hose, but contains millions of “pores” through which substances can pass from one side to the other. Some of them are indeed pores of various sizes; as it has now turned out, they are proteins in the form of tubes that penetrate through the fatty substance of the membrane. In other cases, they are not just pores, but miniature protein machines called pumps; they are able to capture one type of ion and expel them from the cell, while simultaneously capturing other ions inside from the outside. Such pumping requires the expenditure of energy, which the cell ultimately receives in the process of glucose oxidation. There are also pores called canals, which are “valves” that can open and close. What influences lead to their opening or closing depends on the type of pores. Some of them are influenced by membrane potential, others open or close in the presence of certain substances in the internal and external fluid.
The potential difference across the membrane at any moment is determined by the concentration of ions inside and outside, as well as whether the various pores are open or closed. (I said above that potential influences pores, and now I argue that pores influence potential. Let's just say for now that these two things can be interdependent. A more detailed explanation will be given a little later.) Since there are several types of pores and several types of ions, it is easy to understand that the whole system is quite complex. When Hodgkin and Huxley figured it out in 1952, it was a huge achievement. Let us first ask ourselves the question: how is the potential difference created?
Let us assume that at first there is no difference and the concentrations of ions inside and outside are the same. Let us then turn on a pump that removes ions of one type, for example sodium, from the cell, and instead of each removed ion, transfers an ion of another type, for example potassium, inside. The pump itself does not create any potential, since as many positively charged ions are pumped in, the same number are pumped out (sodium and potassium ions carry the same positive charges). But let us now imagine that for some reason a large number of pores of one type have opened, for example potassium pores. Potassium ions will begin to flow through them, and the flow rate through each open pore will depend on the potassium concentration: the more ions near the pore opening, the greater their leakage through the membrane; and since there are more potassium ions inside than outside, there will be more of them coming out than going in. But if more charges come out than come in, the outer space will quickly become electropositive with respect to the inner space. This accumulation of positive charge on the outside will soon begin to counteract the further release of potassium ions from the cell, since like charges repel each other.
Very quickly - before the release of K4 ions leads to a noticeable change in their concentration - the positive charge on the outside will reach a value at which it exactly compensates for the tendency of K+ ions to leave the cell (there are more potassium ions on the inside of the pore, but they are repelled by the outer charge). From this moment on, the movement of charge stops, and we say that the system comes into equilibrium. Thus, the opening of potassium pores leads to the appearance of a potential difference on the membrane with a positive pole on the outside.
But suppose the sodium pores opened instead. By repeating all the reasoning and replacing the words “internal” with “external”, you can easily verify that the result will be exactly the opposite: a negative charge will arise outside. With the simultaneous opening of both types of pores, the result would be a “compromise”. To estimate the magnitude of the membrane potential, we must know the relative concentrations of the two ions and the ratio of the number of open and closed pores for each ion, and then make the appropriate calculations.